The standard inner product

An inner product is a generalization of the dot product. In a vector space, it is a way to multiply vectors together, with the result of this multiplication being a scalar. Let u, v, and w be vectors and alpha be a scalar, then: 1. When restricted to homogeneous polynomials (of the same degree), it is known as the Bombieri inner product. In other circles, it is known as the Fisher inner product.

In mathematics, the dot product or scalar product is an algebraic operation that takes two equal-length sequences of numbers (usually coordinate vectors) and returns a single number. Define Standard inner product. Standard inner product synonyms, Standard inner product pronunciation, Standard inner product translation, English dictionary definition of Standard inner product. See scalar product.

In linear algebra, an inner product space is a vector space with an additional structure called an inner product. This additional structure associates each pair of vectors in the space with a scalar quantity known as the inner product of the vectors. The standard Lorentzian inner product on is given by (1) i. Euclidean n-space, and the dot product is called the standard inner product on Rn.

Find the matrix of the standard inner product on W (obtained by restriction of the dot product on Rª) with respect to the basis S. Please solve (3), (4), and (5), use typefont, and show your work. At this point you may be tempted to guess that an inner product is defined by abstracting the properties of the dot product discussed in the last paragraph.

For real vector spaces, that guess is correct. Une multitude de produits de qualité pour des projets réussis ! Linear Algebra: We define the standard inner product on R^n and explain its basic properties. A cosine formula is given in terms of the inner product and len.

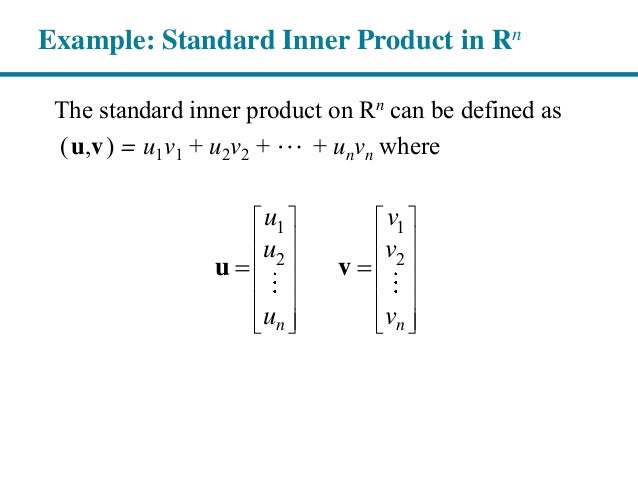
This question is from the book Elements of Differential Geometry. I don not really understand what the question is asking. Note that the outer product is defined for different dimensions, while the inner product requires the same dimension. Find the inner products of the following pairs of functions and state whether they are orthogonal 1. The question is not using the standard inner product.

More generally, an inner product is a function that takes in two vectors and gives a complex number, subject to some conditions. This can be viewed as the product of a "bra" and a "ket". We explain what these mean for standard inner products on. A wide variety of standard inner product options are available to you, such as remy hair.

The inner product "ab" of a vector can be multiplied only if "a vector" and "b vector" have the same dimension. The outer product "a × b" of a vector can be multiplied only when "a vector" and "b vector" have three dimensions.

The Euclidean inner product is the most commonly used inner product in. However, on occasion it is useful to consider other inner products. To generalize the notion of an inner product, we use the properties listed in Theorem 8. A complex vector space with a complex inner product is called a complex inner product space or unitary space. Which of the following vectors in Plie in the subspace W(perpendicular)?

The verification that this gives an inner product relies on standard properties of Riemann integrals. Given that this is a real inner product, I may apply the preceding proposition to produce some useful. Consider P, with the standard inner product.

The vector space Cn has a standard inner product, hu,vi = u∗v. Recall u∗ = uT so another formula is hu,vi = uTv.